How To Calculate The Future Value Of An Ordinary Annuity Using The Formula Explained
In this video we discuss how to calculate the future value of an ordinary annuity using the formula. We go through a few examples, including ones where the compounding periods do not match the payment/deposit periods
Transcript/notes
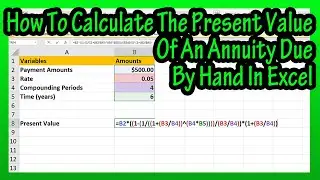
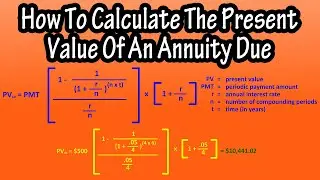
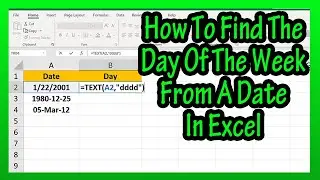
The formula to calculate the future value of an ordinary annuity is future value equals, the payment amount, times the quantity, 1 plus the annual rate divided by the number of compounding periods per year, raised to the number of compounding periods per year times the time in years, minus 1, divided by the quantity, the annual rate divided by the number of compounding periods per year.
Here is the formula with all of the variables listed. And one important note, to use this formula, the interest compounding periods must match the payment periods, for instance, yearly, semi-annually or quarterly. This is extremely important.
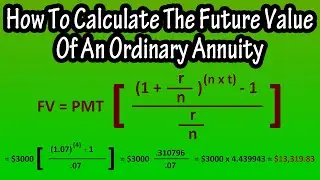
As an example, let’s say that someone deposits $3000 at the end of each year for 4 years, into an investment earning 7% compounded yearly. What is the future value of the annuity?
Plugging into the formula we have future value equals, $3000 times the quantity, 1 plus .07, the decimal value of 7%, divided by 1, raised to the 4 times 1, minus 1, divided by .07, divided by 1.
Here are all of the steps with all of the calculations written out, and we end up with $3000 times 4.439943 rounded off, and this calculates to $13,319.83 rounded off.
Now, as another example, for when the payment periods do not match the compounding periods, let’s say this time, that someone deposits $3000 at the end of each year for 4 years, into an investment earning 7% compounded quarterly. What is the future value of the annuity?
So, the interest compounding periods are quarterly and the payment periods are yearly. We need to get the compounding periods to match the payment periods.
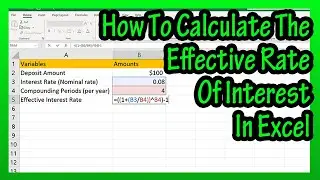
To do this, we need to convert the nominal interest rate of 7% to the effective rate, and the formula for this is, effective annual rate equals, the quantity, 1, plus the annual rate, divided by the number of compounding periods per year, raised to the number of compounding periods per year, minus 1.
Now we can plug into the formula and I have done the calculations, we get the effective rate equals, 7.1859% rounded off.
And now we can calculate the future value of the annuity using the main formula. We have, future value equals, $3000 times the quantity, 1 plus .071859, the er or effective rate, divided by 1 raised to 1 times 4, minus 1, divided by the quantity, .071859, divided by 1. And this calculates to $13,356.54.
If we compare it to the previous problem, the annuity that compounds quarterly has a higher future value, and that makes sense, because of more compounding periods.
One last example, this time someone deposits $500 at the end of each month, again for 4 years, the yearly rate is 7%, but is compounded quarterly. What is the future value of the annuity?
So, again, the compounding periods do not match the payment periods, what we need to ultimately do, is convert the yearly interest rate to a monthly rate, to match the deposit periods.
And to do this, we have to first, convert the interest rate to the effective yearly rate, then second, convert it to the nominal monthly rate.
Here again is the formula to convert it to the effective rate. And here is the formula to convert the effective rate to the nominal rate.
So, first to convert to the effective rate, we have effective rate equals, the quantity, 1, plus .07 divided by 4, raised to the 4, minus 1. And the calculations are on the screen, we get effective rate equals, .071859, same as the last example.
Now for the nominal rate, we have nominal rate equals, 12, the number of compounding periods, it is 12 because we need it to match the monthly deposits, times the quantity, 1 plus .071859, raised to 1 divided by 12, minus 1. I have done the calculations on the screen, and we get nominal rate equals, .069596.
And now we can calculate the future value of the annuity using the main formula. We have, future value equals, $500 times the quantity, 1 plus .069595561, divided by 12, the number of deposit and compounding periods, raised to 12 times 4, minus 1, divided by the quantity, .069595561, divided by 12. Here are all of the calculations on the screen, and this calculates to $27,581.86.
Chapters/Timestamps
0:00 Formula to calculate the future value of an ordinary annuity
0:42 Example 1 how to calculate the future value of an ordinary annuity
1:35 Example 2 when payment deposit periods do not match compounding periods
2:06 How to convert nominal rate to effective rate
2:51 Answer to example 2
3:10 Example 3 monthly payment deposit periods, quarterly compounding periods
4:07 How to convert effective rate to nominal rate
4:59 Answer to example 3