The Finite Difference Method for non-linear differential equations (1D)
This video builds upon my previous video • The Finite Difference Method (1D)
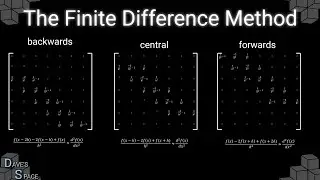
in which I introduced the finite difference method for solving linear ODEs.
When the equations to solve are non-liear direct methods cannot be used as non-linear terms cannot be expressed as a linear combination of neighbouring grid points. Instead iterative methods must be used. I introduce a single iterative method the, modified richardson method.
I visually show how the solution converges from an initial guess to a good approximation of the solution at the grid points.
Future videos in this series will include:
-Solving 2D problems with Dirichlet and Neumann boundary equations
-Solving physics equations such as the heat equation with non-uniform material properties